Paper:
Nonlinear elasticity of rectangular
elastomeric seals
Authors: Nikas, G. K, Sayles, R. S.
Published in: Tribology
International, 2004, 37(8), 651-660
Abstract
Elastomeric seals used in high-pressure hydraulic systems obey complex stress-strain laws when sufficiently strained, which occurs for high sealed pressure and large temperature differentials. Such seals often operate in extreme environments of pressures up to 35 MPa (5000 lb/in2) and temperatures in the range of –60 to +140 ºC. For the elastohydrodynamic numerical analysis of these seals it is imperative that the rubber behaviour under stress is properly described with a suitable stress-strain model, such as the popular Mooney-Rivlin model. Such a nonlinear model is developed and tested in this study to produce important numerical results for the seal performance (contact pressure and width, film thickness, leakage rate and friction). The nonlinear model is then compared with the classic Hookean model of linear elasticity in order to establish the differences of the models and the limits of applicability and validity of each one for a wide range of operating conditions.
Some figures from this work
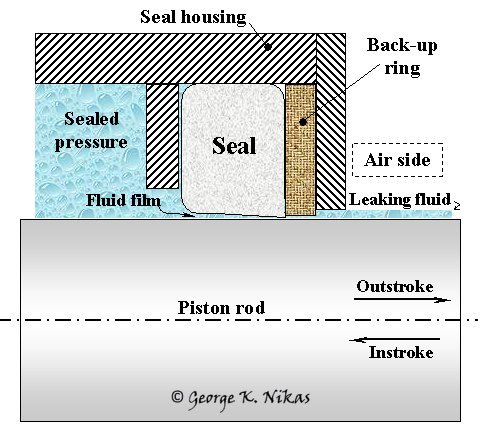
The hydraulic actuator and seal configuration for the model is shown in Fig. 1. The actuator contains fluid under pressure, usually between 0.1 and 35 MPa, and operates between –60 and +140 ºC. The piston rod reciprocates and fluid leaks under the elastomeric seal, past the (optional) back-up ring, and escapes to the air side of the system.

Fig. 1. Configuration for the seal model.
Figure 2 below shows an example of the computed elastohydrodynamic contact pressure between the elastomeric rectangular seal (which has slightly curved corners) and the piston rod of the actuator. The difference in the result between the Mooney-Rivlin type model of nonlinear elasticity developed in the paper and the classic Hookean model of linear elasticity is clear to see. The absolute value of the contact pressure depends not only on the sealed pressure, which is transferred directly to the seal via its wet side, but also on the initial interference as well as the operating temperature, which affects the dimensions of all elements of the system, especially those of the rubber seal, which normally has a high thermal expansion coefficient.

Fig. 2. Elastohydrodynamic contact pressure (pcyl = 27.6 MPa = 4000 lb/in2).
Figure 3 below shows the corresponding average film thickness at the rod-seal contact and the differences when this is computed with the two elasticity models. As can be seen, the figure covers a wide range of sealed pressures and operating temperatures. The results reveal that the film thickness difference between the two models is rather significant at high sealed pressure and high temperature (simultaneously) but is otherwise small.
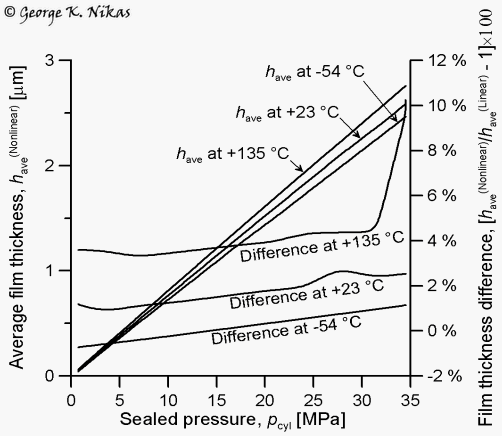
Fig. 3. Average film thickness at the rod-seal contact.
Figure 4 below shows the corresponding leakage of the seal (mass leakage rate), again for the widest practical range of operating conditions. According to the simulation, the leakage difference between the two elasticity models is greater at high pressure and temperature (up to about 15 %) but is otherwise low.
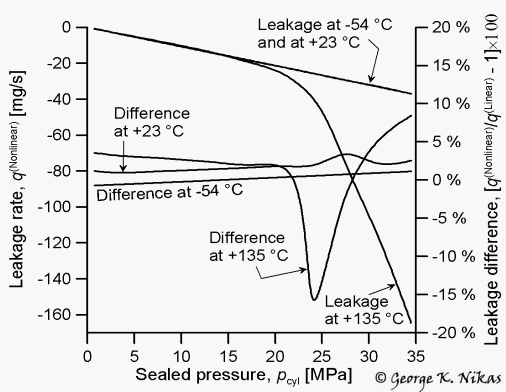
Fig. 4. Leakage of the seal.
The results should not be considered in absolute value but only as an indication of how things are expected to be. There are many hidden variables in this system, such as the wear rate of the seals during service, the history of the variation of the sealed pressure, which will affect the wear of the seal, the ageing of the seal with large temperature variations (normal for example in aircraft actuators), the swelling of the seal owing to fluid uptake, etc. These are difficult to be taken into account during a general simulation. Moreover, there is ambiguity about the leakage (and friction) results at high temperature because of possible fluid vaporization, which affects the contact condition at the rod-seal contact and is also difficult to be measured experimentally as the fluid may leak without being detected.
For more information in this research area, please see the author's related sealing project.